Techno-Sublime

Benoit Mandelbrot, who died in 2010, was a Polish-born, French-educated mathematician who flourished and became famous in America. His special genius was his ability to disregard disciplinary boundaries and find a common pattern underlying disparate phenomena. From adolescence on he was possessed by an urgent desire to invent a mathematical object that would transform the way we look at the world. By most accounts, certainly his own, he was successful: ‘What shape,’ he asks,

is a mountain, a coastline, a river, or a dividing line between two river watersheds? What shape is a cloud, a flame, or a welding? How dense is the distribution of galaxies in the universe? How can one describe – to be able to act upon – the volatility of prices quoted in financial markets? How to compare and measure the vocabularies of different writers? … These questions, as well as a host of others, are scattered across a multitude of sciences and have been faced only recently … by me.

His achievement was to give mathematical form to the feature all these shapes have in common: namely that each exhibits a similarity with itself at different scales. For example, the chart of daily price variation of a commodity over a year will be statistically similar to the chart of its minute by minute variation over the course of a day; the nature of this similarity is a measure of the chart’s intrinsic ‘roughness’.

Mandelbrot’s hero and scientific model, and the dedicatee of his memoir, is Johannes Kepler, ‘who brought ancient data and ancient toys together and founded science’. Kepler’s toys were the ellipses that replaced circles as the true paths of the planets. The wish to emulate Kepler fuelled the search for the mathematical structures Mandelbrot was to call fractals, the founding concept of his science of roughness. What are fractals? The short answer is mathematical models of self-similarity and self-resemblance: objects whose parts mimic the whole. Natural examples abound: a cauliflower is made up of florets, or miniature cauliflowers, each of which in turn is composed of smaller florets, and so on. A tree – a trunk and two branches – repeats itself: each branch behaves like the trunk and forms two branchlets, and so on. The peaks on a mountain mimic its overall jaggedness, the rocks on each peak in turn mimic them, and so on. One gets the idea.

The memoir opens with a family photograph, a group portrait taken at a dinner party in June 1930 in the house in the Warsaw ghetto where Mandelbrot was born, every person at which ‘deeply affected either my blood or my spirit’. In the place of honour sits the mathematician Jacques Hadamard, ‘arguably the greatest mathematician in France at that time’. Far left is Szolem Mandelbrot, Benoit’s mathematician uncle, who was to play an important role in his scientific life. The dinner party was held to celebrate Szolem being chosen as one of four professors to represent France at the First Congress of Mathematics of the Soviet Union. Two other French mathematicians are present, Arnaud Denjoy and Paul Montel, who had supervised Szolem’s PhD thesis. The others at the dinner table are Benoit’s father (second from left, referred to throughout as ‘Father’), his grandfather Szlomo, the white-bearded patriarch of the family who spoke only Yiddish, and his cousin Leon, the editor of a Polish-language Jewish newspaper. At the back stand the only woman, his aunt Helena Loterman, who looked after her father, Szlomo, and her husband, both of whom ‘vanished in the Holocaust’. Not in the photo is the six-year-old Benoit, his younger brother, Léon, or their mother (always ‘Mother’).

Shortly after the photograph was taken the Depression hit and Father left for Paris to save his wholesale clothing business. In 1936 he decided the whole family must move to Paris, to a two-room flat in Belleville, then a slum quarter. Within a year, after intense drilling from Mother, Mandelbrot passed the dreaded certificat d’études élémentaires and entered a lycée, where he was soon ‘way ahead, reading and dreaming on my own’: dreaming over the strange shapes in an out-of-date mathematical textbook and devouring an obsolete multi-volume Larousse Encyclopedia Father had lugged home. Father and son would go on educational walks across Paris; a sign reading Ecole Polytechnique in faded gold letters prompted the fatherly dream that Benoit might one day attend that illustrious institution. In 1939, Father moved the family to Tulle in the South-West, in the ‘dirt-poor hills of unoccupied Vichy France’.

Tulle too became dangerous after the family’s secret protector, the politician Henri Queuille, lost influence. Mandelbrot acquired a false ID, moved around taking odd jobs, became an apprentice in a tool-making shop in Périgueux and ended up in Lyon. There, as a student on the ‘taupe’, the course that prepared students likely to qualify for one of the grandes écoles, Mandelbrot became aware of his mathematical gift. Over the years, the lycée teachers who coached students on the maths taupe had devised speed trials, problems requiring long, complex calculations that students were not expected to finish. Repeatedly, Mandelbrot would raise his hand: ‘Monsieur, I see an obvious geometric solution.’ Repeatedly, he would be right.

In August 1944, the Allies landed in southern France and Mandelbrot returned to Paris. He succeeded brilliantly in the exams for the Ecole Normale Supérieure and the Ecole Polytechnique, and once again his geometrical gift was clear: no other candidate finished the triple integral question at the end of the Polytechnique papers. His baffled teacher asked how he did it: ‘I saw that it is the volume of the sphere. But you must first change the given co-ordinates to the strange but intrinsic co-ordinates I thought the underlying geometry suggested.’ Mandelbrot could choose between the Normale (to study pure mathematics/theoretical physics) and the less prestigious Polytechnique (applied mathematics/engineering). A family council, of Szolem, Father and a cousin (a distinguished chemist), was convened. For Szolem, the choice was clear: if Benoit was to be a serious mathematician, it had to be the Normale. The cousin added little; Father, a strategic survivor of many upsets, thought the Polytechnique’s training in science/engineering more use in a rapidly changing world. Benoit went with the Normale, but by the end of his first day he knew it was ‘absolutely the wrong place for me’. The Bourbaki movement led by André Weil, which saw mathematics as the study of abstract sets unconnected to anything outside itself, was about to take over the Normale. Mandelbrot abhorred the movement, called it a ‘cult’, considered its approach to mathematics ‘positively repellent’, and Weil himself (arguably, one of the century’s leading mathematicians) his ‘nemesis’. The next morning he switched to the Polytechnique.

But his years there left him frustrated: his ‘Keplerian dream remained stuck in a holding pattern’. One of his professors suggested fluid mechanics and Caltech as the best place to study it. Intrigued by aeronautics, and ignoring Szolem’s disapproval, he spent the next two years in Pasadena. Caltech then boasted a slew of world-changing scientists, and in particular Max Delbrück, who was orchestrating the birth of molecular biology – ‘exhilarating proof that someone with my bent might have a chance after all’. But his work there led nowhere and Mandelbrot returned to France, to serve his compulsory year in the military and find a job. Father, scouring newspaper ads, discovered that Philips was looking for an English-speaking alumnus of the grandes écoles to work in Paris on the development of colour television. Mandelbrot spent an enjoyable two years as an electronic research engineer.

His mathematical reading, meanwhile, was going nowhere. A frustrated Szolem gave him ‘a verbal lashing’, and demanded that he settle on a thesis topic. When he visited his uncle, he usually asked for something mathematical to read on the long metro ride back to Belleville. On one occasion, Szolem reached into his wastepaper basket: ‘Take this reprint. That’s the kind of silly stuff only you can like.’ It was a review of a book by George Kingsley Zipf, an eccentric Harvard scholar, whose eponymous law concerns word frequencies in any language. For written English, the most frequent word (rank 1), ‘the’, will occur approximately twice as often as the second most frequent word (rank 2), ‘of’, three times as often as the third most frequent, ‘and’ etc. On the journey home Mandelbrot was smitten. Where did such a law come from? Why did it resemble laws in statistical mechanics and information theory? What could possibly link the statistics of word frequencies and the behaviour of molecules?

Zipf’s law was an example of a kind of law that occurs throughout physics, known as a power law. A power law is a relationship between two quantities, where one quantity varies as a power of the other. For example, a person’s weight varies as a cube of their height (power = 3); the gravitational force between two bodies varies as the inverse square of the distance separating them (power = minus 2). And, by Zipf’s law, the frequency of a word varies inversely as its rank (power = minus 1). The principle applies not just to word frequencies but to the sizes of islands, populations of cities, weeks on bestseller lists and much else; in the 1890s, the Italian economist Vilfredo Pareto had observed it in the distribution of personal incomes in Italy. In all these cases, a relatively small number of items – commonest words, richest individuals – account for a large chunk of the total, with the rest diminishing very slowly down to nothing: graphically, unlike the familiar bell curve of random variation from an average, the distribution curve of frequencies has a large hump with a very long tail. The encounter with Zipf’s law was ‘the first of many Kepler moments’ in Mandelbrot’s life. He remained obsessed by power laws for more than a decade and their ‘long tails’ would resurface in another Kepler moment. The law, now transmuted into the Zipf-Mandelbrot law, became his topic. His dissertation, hurriedly assembled, poorly organised, in a field that didn’t yet exist, attracted little attention; but it meant he could apply for postdoctoral positions.

Fortune smiled. Mandelbrot was now to come into close contact with Keplerian giants: a year’s postdoc at MIT where Norbert Wiener presided, then a year at Princeton’s Institute of Advanced Study as an assistant to John von Neumann. A chance meeting with Robert Oppenheimer led to an invitation to talk about the Zipf-Mandelbrot law at the Institute. The talk fell flat, people fell asleep, and a distinguished historian of mathematics stood up and declared he hadn’t understood a word. Mandelbrot was paralysed, but Oppenheimer came to the rescue, succinctly summarising the lecture’s content, and was followed by von Neumann, who added to its mathematics. After Princeton came a return to France, marriage to Aliette Kagan, a two-year stint in Geneva at the invitation of Piaget, the birth of a son, Laurent, and a job at the University of Lille. But he wasn’t content, whence his chapter heading, ‘An Underachieving and Restless Maverick Pulls up Shallow Roots, 1957-58’. He moved back to America to begin ‘My Life’s Fruitful Third Stage’.

A summer job in New York at IBM turned into a 35-year stay. The company allowed him virtually free rein as a research scientist at large. He lectured whenever invited and his ideas attracted enough attention for him to take two years’ leave from IBM to present his work at MIT and Harvard. Harvard was the scene of a second, more significant Kepler moment. Invited to speak about his work on the distribution of personal incomes and popping in to see the professor whose group he was to address, he was taken aback: there on a blackboard was the very diagram he was about to draw in his class. But it related to a study of daily cotton prices, a subject before this moment – and that was the revelation – Mandelbrot would have considered remote from his own topic that day. Subsequent study revealed that the number of spikes on the graph greatly exceeded the predictions made by the only known mathematical model of commodity prices, in a 1900 doctoral thesis by Louis Bachelier. Bachelier had assumed two ‘facts’: that prices at any given point in time are independent of their predecessors (like coin tosses); and that they vary randomly according to the familiar bell-shaped curve. For Mandelbrot the distribution of personal incomes and cotton prices had some deep common features, and once again, the phenomenon of long tails was in evidence. He set to work to find the flaw in Bachelier’s assumptions. But the moment was against him: mathematical economists were rediscovering Bachelier’s thesis, blithely assuming its ‘facts’ were correct, and working them into models of market behaviour, price volatility, risk profiles, portfolio theory and the Black-Scholes formula for pricing derivatives.

Lecturing at MIT and Harvard raised Mandelbrot’s profile and the possibility of a professorship at one or the other, but nothing was offered and he returned to the comfortable world of IBM. He went to ground, working for the next ten years on the yet to be crystallised concept of fractals, slowly figuring out its mathematics. He continued to think about natural shapes. How long, he asked, is the coastline of Britain? The answer: it depends, the smaller your tape measure the longer the coastline. Seen from a satellite, a bay is smooth; seen from a low-flying plane, inlets and promontories are revealed; seen from a boat cruising the shoreline, each of these inlets is seen to be made up of smaller formations, and so on. The shape of the coastline repeats its characteristic roughness at ever smaller scales, its length increasing down to the level of individual sand grains. (Once he had the concept, he could assign a number – its fractal dimension – to a coastline. Britain’s western coastline has a fractal dimension 1.25; the much smoother coastline of South Africa about 1.02.)

Meanwhile, he was piecing the fractal concept together. He created the fractal dimension from a concept formulated fifty years earlier by Felix Hausdorff and found the required long-tailed probability curves in, of all places, the Ecole Polytechnique. They were what his old professor there, Paul Lévy, worked on. By the early 1970s he was able to announce the birth of fractals, both the concept and the name, which he coined from the Latin fractus. Les Objets fractals: Forme, hasard et dimension appeared in 1975, and was followed by an expanded English version, Fractals, and the richly illustrated Fractal Geometry of Nature. The year 1979-80 he spent at Harvard was his annus mirabilis. He was invited to teach a course in the mathematics department, began new collaborations in physics and pure mathematics and, the final wonder, discovered/invented the extraordinary object known as the Mandelbrot set.

Magnifications of the Mandelbrot set

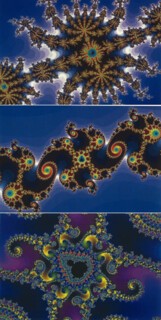
‘The most complex mathematical object in existence’, the Mandelbrot set is a two-dimensional figure whose coils, seahorse shapes and blobs rimmed by jewel-like clusters of islands defy any coherent description. It is made up of infinitely many resemblances of itself, no two exactly alike, which appear from its depths when one zooms in and magnifies any part of it. The set has attracted countless admirers, from engineers, chaos theorists and artists, to Platonist mathematicians, and others for whom it serves as a techno-sublime mandala.

The memoir illustrates a fragment of it, along with a scattering of black and white images, mostly fractal simulacra of mountains, snowflakes, clouds and the like, as well as a set of colour illustrations of fractal-inspired art and mathematical constructs. The Mandelbrot set has become a visual icon of fractal complexity and chaos theory, an object of deep mathematical research as well as philosophical speculation. (A recent student of mine included a 15-page meditation on it in her PhD dissertation.) Its infinite complexity and dizzying, ever-changing depths notwithstanding, the object results from an astonishingly simple algorithm (symbol-averse readers might want to skip the next paragraph).

Imagine you could add and multiply points in a plane, with the result forming another point (you can if you identify the points with complex numbers). This allows you to form functions such as f(x) = c + x², where c is a fixed and x a variable point: if one inputs a point p for x, the function will output the point c + p². One now repeats the function by using its output as a new input, starting with x = 0. The result will be a series of points, first c when x is 0, followed by c + c² when x is now c, then c+ (c + c²)², and so on, generating an infinite path of points in the plane. If the path spirals off to infinity mark the point c white, otherwise mark it black. Carry out the entire procedure for each point c in the entire plane. The resulting black image on a white background is the Mandelbrot set. Note that no human being can draw the image, only a computer can. (The French mathematician Gaston Julia studied iterations of such quadratic functions earlier in the century; if computer graphics had existed then, the object would today be called the Julia set.)

After his year of marvels, Mandelbrot’s achievements were increasingly recognised. His fame as the father of fractals grew; universities, technical institutes and learned societies honoured him with numerous awards and prizes. He was made a professor in the Yale mathematics department, and ultimately, to his immense pride, promoted to the Sterling professorship, ‘the university’s highest rank’. He continued to collaborate on a variety of topics, and to publish such monographs as Fractales, hasard et finance and, at 80, Fractals and Chaos: The Mandelbrot Set and Beyond.

He died suddenly as he was finishing this memoir, having realised his heroic dream of revealing the mathematical order behind the geometry of rivers, stock prices, clouds, coastlines, word frequency and clusters of galaxies. In a touching afterword, Michael Frame, a Yale professor who collaborated with him, mourns the hole left by his absence; he ends with an aperçu from Mandelbrot’s last major talk, just before his death: ‘Bottomless wonders spring from simple rules … repeated without end.’ A profound truth, and nowhere more true (or profound) than in mathematics, whose endless wonders spring from repeating the simplest of all possible rules: leave a mark.